public static double s09ab(
	double x,
	out int ifail
)

Public Shared Function s09ab ( _
	x As Double, _
	<OutAttribute> ByRef ifail As Integer _
) As Double

public:
static double s09ab(
	double x, 
	[OutAttribute] int% ifail
)

static member s09ab : 
        x : float * 
        ifail : int byref -> float 

s09ab returns the value of the inverse circular cosine, $\arccos x$; the result is in the principal range $\left(0,\pi \right)$.

s09ab calculates an approximate value for the inverse circular cosine, $\arccos x$. It is based on the Chebyshev expansion where $\frac{-1}{\sqrt{2}}\le x\le \frac{1}{\sqrt{2}}\text{, \hspace{1em} and \hspace{1em}}t=4x^2-1$.

For $x^2\le \frac{1}{2}\text{, \hspace{1em}}\arccos x=\frac{\pi }{2}-\arcsin x$.

For $-1\le x<\frac{-1}{\sqrt{2}}\text{, \hspace{1em}}\arccos x=\pi -\arcsin \sqrt{1-x^2}$.

For $\frac{1}{\sqrt{2}}<x\le 1\text{, \hspace{1em}}\arccos x=\arcsin \sqrt{1-x^2}$.

For $\left|x\right|>1\text{, \hspace{1em}}\arccos x$ is undefined and the method fails.

Abramowitz M and Stegun I A (1972) Handbook of Mathematical Functions (3rd Edition) Dover Publications

Errors or warnings detected by the method:

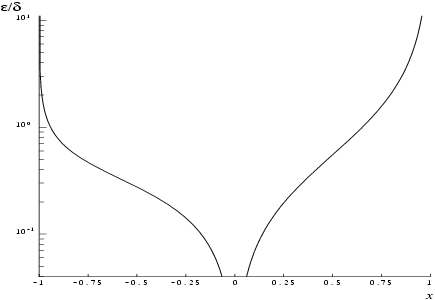
If $\delta$ and $\epsilon$ are the relative errors in the argument and the result, respectively, then in principle The equality should hold if $\delta$ is greater than the machine precision ($\delta$ is due to data errors etc.), but if $\delta$ is due simply to round-off in the machine it is possible that rounding etc. in internal calculations may lose one extra figure.

The behaviour of the amplification factor $\frac{x}{\arccos x\sqrt{1-x^2}}$ is shown in the graph below.

In the region of $x=0$ this factor tends to zero and the accuracy will be limited by the machine precision. For $\left|x\right|$ close to one, $1-\left|x\right|\sim \delta$, the above analysis is not applicable owing to the fact that both the argument and the result are bounded $\left|x\right|\le 1$, $0\le \arccos x\le \pi$.

In the region of $x\sim -1$ we have $\epsilon \sim \sqrt{\delta }$, that is the result will have approximately half as many correct significant figures as the argument.

In the region $x\sim +1$, we have that the absolute error in the result, $E$, is given by $E\sim \sqrt{\delta }$, that is the result will have approximately half as many decimal places correct as there are correct figures in the argument.

None.

None.

This example reads values of the argument $x$ from a file, evaluates the function at each value of $x$ and prints the results.