NAG Library Routine Document
S09AAF
1 Purpose
S09AAF returns the value of the inverse circular sine, , via the function name. The value is in the principal range .
2 Specification
| REAL (KIND=nag_wp) S09AAF |
| INTEGER |
IFAIL |
| REAL (KIND=nag_wp) |
X |
|
3 Description
S09AAF calculates an approximate value for the inverse circular sine,
. It is based on the Chebyshev expansion
where
and
.
For .
For .
For is undefined and the routine fails.
4 References
Abramowitz M and Stegun I A (1972) Handbook of Mathematical Functions (3rd Edition) Dover Publications
5 Parameters
- 1: X – REAL (KIND=nag_wp)Input
On entry: the argument of the function.
Constraint:
.
- 2: IFAIL – INTEGERInput/Output
-
On entry:
IFAIL must be set to
,
. If you are unfamiliar with this parameter you should refer to
Section 3.3 in the Essential Introduction for details.
For environments where it might be inappropriate to halt program execution when an error is detected, the value
is recommended. If the output of error messages is undesirable, then the value
is recommended. Otherwise, if you are not familiar with this parameter, the recommended value is
.
When the value is used it is essential to test the value of IFAIL on exit.
On exit:
unless the routine detects an error or a warning has been flagged (see
Section 6).
6 Error Indicators and Warnings
If on entry
or
, explanatory error messages are output on the current error message unit (as defined by
X04AAF).
Errors or warnings detected by the routine:
The routine has been called with an argument greater than in absolute value; is undefined and the routine returns zero.
7 Accuracy
If
and
are the relative errors in the argument and result, respectively, then in principle
That is, a relative error in the argument
is amplified by at least a factor
in the result.
The equality should hold if is greater than the machine precision ( is a result of data errors etc.) but if is produced simply by round-off error in the machine it is possible that rounding in internal calculations may lose an extra figure in the result.
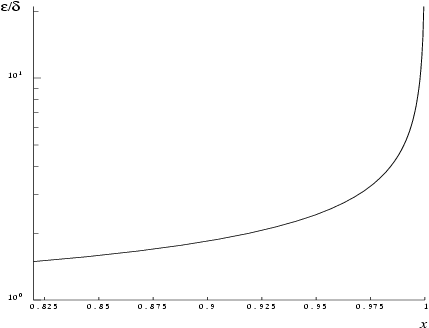
This factor stays close to one except near where its behaviour is shown in the following graph.
Figure 1
For
close to unity,
, the above analysis is no longer applicable owing to the fact that both argument and result are subject to finite bounds, (
and
). In this region
; that is the result will have approximately half as many correct significant figures as the argument.
For the result will be correct to full machine precision.
None.
9 Example
This example reads values of the argument from a file, evaluates the function at each value of and prints the results.
9.1 Program Text
Program Text (s09aafe.f90)
9.2 Program Data
Program Data (s09aafe.d)
9.3 Program Results
Program Results (s09aafe.r)