S13ACF returns the value of the cosine integral
via the routine name where
denotes Euler's constant.
For
it is based on the Chebyshev expansion
For
where the value of
is given in the
Users' Note for your implementation,
where
and
,
.
For
,
to within the accuracy possible (see
Section 7).
If on entry
or
, explanatory error messages are output on the current error message unit (as defined by
X04AAF).
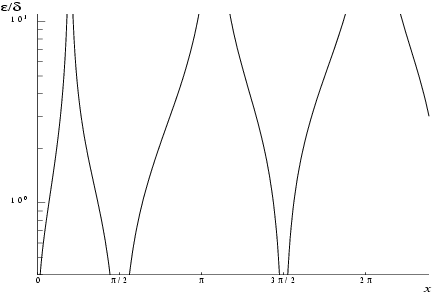
If
and
are the absolute and relative errors in the result and
is the relative error in the argument then in principle these are related by
That is accuracy will be limited by
machine precision near the origin and near the zeros of
, but near the zeros of
only absolute accuracy can be maintained.
The behaviour of this amplification is shown in
Figure 1.
Figure 1
For large values of
,
therefore
and since
is limited by the finite precision of the machine it becomes impossible to return results which have any relative accuracy. That is, when
we have that
and hence is not significantly different from zero.
None.